Background

We have five exercises on discrete maps. Before working on this one, you should first do the Logistic Map exercises.

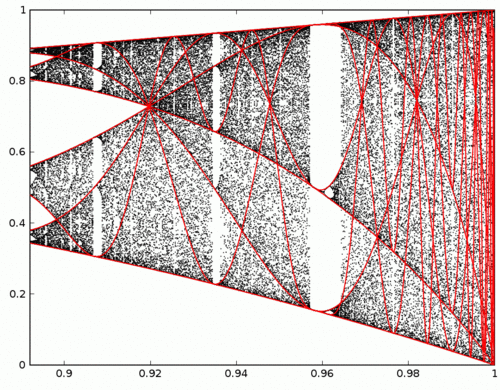
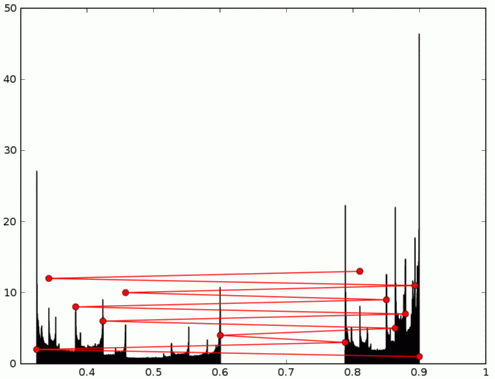
Chaotic dynamical systems will stretch and fold the state space. In Hamiltonian systems, this stretching and folding preserves volume in phase space, and the natural weighting of all points in phase space is equal (Liouville's theorem). In more general chaotic dynamical systems with dissipation and forcing, the long-time behavior lies on an attractor: points off of the attractor do not contribute to the time-average behavior, and points on the attractor are weighted by an invariant measure or probability density in state space.

In this problem we explore the invariant measure for the logistic map, which goes chaotic through a period doubling cascade. The chaotic region of this map exhibits a complex, textured structure with a series of sharp internal boundaries. In this exercise, we attempt to understand this structure.

Learning Goals

Science: You will learn about Chaos, discrete maps, and invarient measures.

Computation: You will learn about iterative algorithms, and the evolution of probability measures under such maps.

Procedure

• Download the file ChaosLyapunovHints.py and rename it "ChaosLyapunov.py".
• Open this file in a text editor (kate or emacs -- we recommend against using kedit) or load it into the IPython dashboard (started with ipython notebook --pylab inline.
• Open a terminal window, move to the correct directory and start ipython, or click on the notebook in the dashboard. You will find it convenient to start python with the --pylab flag -- ie type ipython --pylab or ipython notebook --pylab.
• Follow the directions in Invariant Measure Exercise.