Background

We have five exercises on discrete maps. Before working on this one, you should first do the Logistic Map exercis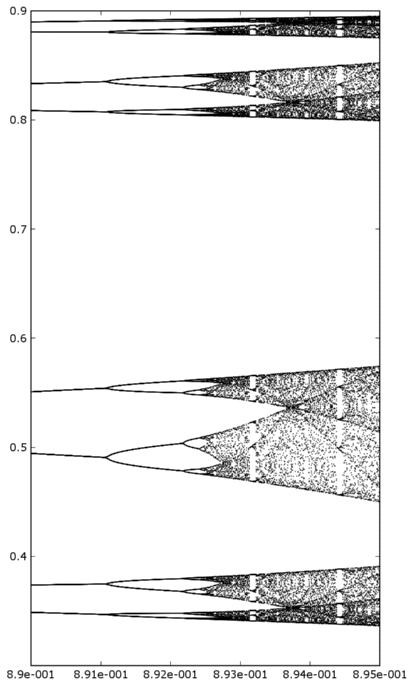es. There are many strange sets that emerge in science. In statistical mechanics, these sets arise at continuous phase transitions where self-similar spatial structures arise. In chaotic dynamical systems, the attractor (the set of all states occupied at long times, after the transients have disappeared) is often very strange. These sets are often tenuous and jagged, with holes on all length scales.

We often try to characterize these sets with a fractal dimension. The dimensions of two extremely different sets, however, can be the same: the path exhibited by a random walk in high dimensions is two--dimensional, just like the surface of a sphere. If the fractal dimensions are different, though, the sets are different.

There is more than one way to define a dimension for a set. This exercise will calculate the capacity dimension (originally called the Hausdorff dimension) and the information dimension (related to the entropy). To generate our strange set, we use a system that both has a strange attractor and is at a continuous phase transition: the logistic map at the period doubling onset of chaos.

Learning Goals

Science: You will learn about Chaos, discrete maps, and fractal dimensions.

Computation: You will learn about iterative algorithms, and the evolution of probability measures under such maps.

Procedure

• Download and have a quick glance at Brief notes on universality, fractal dimensions, and the renormalization group.
• Download the file ChaosLyapunovHints.py and rename it "ChaosLyapunov.py".
• Open this file in a text editor (kate or emacs -- we recommend against using kedit) or load it into the IPython dashboard (started with ipython notebook --pylab inline.
• Open a terminal window, move to the correct directory and start ipython, or click on the notebook in the dashboard. You will find it convenient to start python with the --pylab flag -- ie type ipython --pylab or ipython notebook --pylab.
• Follow the directions in Fractal Dimensions Exercise